Optimal Bowling
If you want to bowl a strike, the optimal place is almost certainly inside a bowling alley, although with a little luck any establishment uphill from one could also work.

If you want to bowl a strike, the optimal place is almost certainly inside a bowling alley, although with a little luck any establishment uphill from one could also work.
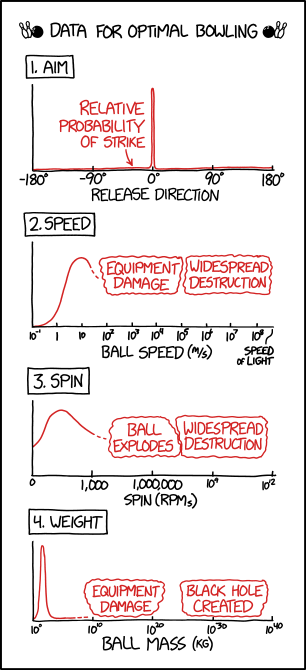
This series of line graphs purports to advise players on how to improve their odds of achieving a strike in the sport of bowling – presumably ten-pin bowling, the most popular version of the sport in the United States. Among the parameters being measured — those being angle of throw, throwing speed, spinning speed, and weight of the ball — all four graphs encompass a range far larger than would be useful for reference by a bowler. The latter three in particular are on logarithmic scales, leading up to values that are impossible for a human to achieve.[citation needed]
The first line graph indicates that a bowler has the greatest chance of achieving a strike by aiming the ball directly at the pins, with the chance of a strike decreasing rapidly as the ball is aimed to the left or the right. The closer you aim to the pins, the more likely it is you hit them.[citation needed] While a novice bowler may have difficulty achieving a 0° angle roll, their roll would still not come close to a -90° or 90° angle (due left or due right), much less a -180° or 180° angle (which, in either case, would be the opposite direction from the pins). Unlike with the other graphs, it is physically possible for a bowler to aim the ball at any angle, albeit not permissible under bowling rules; aiming the ball at an angle which deviates significantly from 0° would most likely cause the ball to end up in the gutter, while more violent or wildly aimed actions could create a risk of the ball going into one of the other lanes or missing the lanes entirely, which could annoy, anger, or even endanger other bowlers and employees of the bowling alley.
The second graph indicates that a bowler has the greatest chance of achieving a strike by throwing the ball about 5–20 m/s (11–45 mph, 18–72 kph), with the chance of a strike decreasing as the speed is increased or decreased. Most bowlers cannot throw more than 45 m/s (100 mph or 160 kph).[citation needed] According to the graph, any throw faster than 100 m/s would cause equipment damage, and then widespread destruction several orders of magnitude later. (Possibly a reference to Relativistic Baseball.) The graph ends at the speed of light, as it is physically impossible to throw anything faster.
The third graph concerns the rotational speed of the ball. The "ball explodes" section is a reference to one of Randall's favorite equations, which is that an object cannot spin faster than the square root of its specific tensile strength. Spinning the ball any faster than this limit would cause the bowling ball to lose its structural integrity and explosively disintegrate. At particularly high speeds, the material of the ball would be flung outwards at a significant fraction of the speed of light, causing, as in the second graph, widespread destruction (possibly a reference to One-Second Day.)
The fourth graph in this comic illustrates a bowler's probability of a strike with a ball whose mass ranges from 100 kg (2.2 pounds) to close to 1010 kg (over 22 billion pounds), and continues by indicating that balls even larger than that would cause "equipment damage" (up to 1020 kg) or the creation of a black hole (starting from around 1025 kg and up). In reality, a ball would be very likely to cause equipment damage at much lower masses than 1010 kg.[citation needed] The last entry on the x-axis of this graph is 1040 kg, which is about 5 billion times the mass of the Sun. The United States Bowling Congress requires all bowling balls to weigh no more than 16 pounds (that is, a mass of no more than 7.257 kg), with no minimum weight. Hence, if the x-axis of the graph ran from, say, 0 to 8 kg, the graph might actually impart some useful information.
The title text continues the trend of providing unhelpful information by stating that the optimal place to stand when trying to bowl a strike is inside the bowling alley, but mentions the possibility of "any establishment uphill from one" working, with a little luck. This suggests the possibility of rolling the bowling ball downhill, in to the bowling alley and the pins, such as in Curious George.